NAME:_________________________________________
Take-home Final
MTH 112
TR 12:00-1:50
Sarah Enoch
Instructions:
1. Due Date: Wednesday, June 11 by 5PM
Drop off in the math office: give to secretary to put into Sarah Enoch’s
box.
2. Show your work!
I did not leave space on the test for you to do
your work, so you must use separate paper. Do your work neatly
and turn it in with the test. For some problems I left a space
for the answers. Please put your answers in those blanks.
However, that is not a substitute for showing your work! If
you fill in the blanks, but do not give me the separate
sheet with your work on it you will not receive full credit. If you
solve the entire problem without writing anything down,
at least write an explanation of how you solved the problem.
3. Do not get help from tutors.
_______________________________________________________________________________________
1. In the Place de la Concorde in Paris there is
a giant Ferris wheel that was erected for the turn of the millennium.
The Ferris wheel is 60 meters high (for this problem, assume that
the height does not include the base). If it takes
1 minute to get from the bottom to the very top, what is the linear speed
(in meters per second)? What is the angular
speed (in radians per second)?
Linear speed=__________________ angular speed=____________________
------------------------------------------------------------------------------------------------------------------
2. Within one period of a sinusoidal function a minimum
occurs at (-3,-1) and a maximum occurs at (3,5). Find a
sinusoidal function of the form y=Asin(ωx-φ)+B that fits the given
data.
Y= ____ sin( ______ * x - ______ ) + ______
---------------------------------------------------------------------------------------------------------------
3. Show that
i. sin(4x) = (cosx)(4sinx-8sin3x)
ii. tan(x) + cot(x) – sec(x)csc(x) = 0
-----------------------------------------------------------------------------------------------------------------
4. a) Plot the point (-5, 7π/6) in polar coordinates, then
convert to rectangular coordinates and plot that point in
rectangular coordinates.
b) Plot the point (-2, 5) in rectangular coordinates, then convert
to polar coordinates and plot that point in polar
coordinates.
-----------------------------------------------------------------------------------------------------------------
5. i. The following function is in rectangular coordinates.
Convert to polar coordinates. Do not try to solve for r.
y = 2x2 + 3x – 4
ii. The following function is in polar coordinates.
Convert to rectangular coordinates. Do not try to solve for y.
r = cos θ + sin θ
-------------------------------------------------------------------------------------------------------------------
6. Just in time for summer vacation Charlie
Brown has made a (not so perfect) kite. The one thing he did get
right was making the cross-sections perpendicular to each other.
Below are two sides and two angles from his kite.
Fill in all the missing information asked for below. (Hint: AB=12in
means the line between points A and B is 12 inches long).
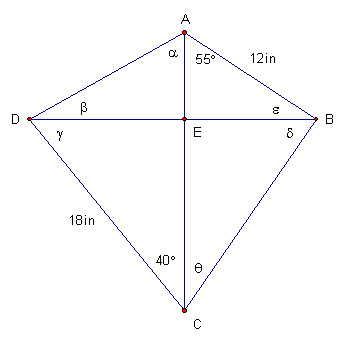
AD=
α= Area of Kite=
CB=
β=
AC=
γ=
DB=
δ=
θ=
ε=
---------------------------------------------------------------------------------------------------------------
7. The lengths of four sides and two angles have
been given below. Solve the upper triangle constructed with
the points A, D, and E.

a =________ α =_________
b =________ β =_________
c =________ γ =_________
Area of Triangle ADE = ______________
---------------------------------------------------------------------------------------------------------------------
8. Find the polar equation that corresponds to the
graph below. Give an explanation for each value you put in your function
(e.g. if there is a 2 in your function, explain how it affects the function).
r =____________________________
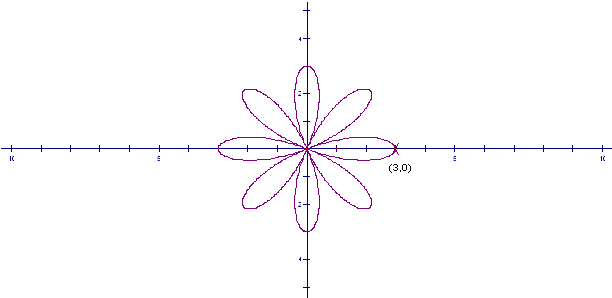
Could you find a polar equation that graphs a rose with 6 sides? If
yes, give an equation that satisfies the condition. If no, why not?


